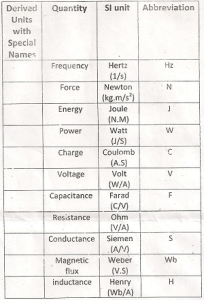
Engineering Mechanics Practice Problems
(REE – Apr. 2005)
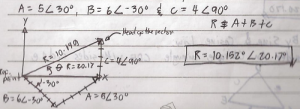
1. Two boys B1 and B2 act at a point. The magnitude of B1 is 8 lbs and its direction is 60 degrees above the horizontal axis in the first quadrant. The magnitude of B2 is 5 lbs and its direction is 53 degrees below the horizontal axis in the fourth quadrant. What is the magnitude of the resultant force?
A. 7.0 lbs
B. 6.9 lbs
C. 7.6 lbs
D. 8.6 lbs
(REE – Apr. 2007)
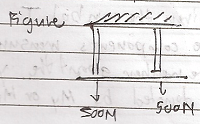
2. Find the resultant value of two 10-lb forces applied by the two ladies at the same point when the angle between the forces is 30 deg. Use any convenient scale.
A. R = 20 lbs
B. R = 19.3 lbs
C. R = 15 lbs
D. R = 23 lbs
(REE – Sep. 2005)
3. Two 10-lb weights are suspended at opposite ends of a rope which passes over a light frictionless pulley. THe pulley is attached to a chain which goes to the ceiling. What is the tension in the rope?
A. 8 lbs
B. 10 lbs
C. 12 lbs
D. 20 lbs
(REE – Sep. 2001 / Sep. 2002 / Apr. 2005)
4. A safe weighing 600 lb is to be lowered at a constant speed down skids 8 ft long from a truck 4 ft high. If the coefficient of sliding friction between safe and skids is 0.30, whaat will you do with the safe? (REE – Sep. 2001)
A. nothing
B. hold back
C. push down
D. lift safe
How great a force parallel to the plane is needed? (REE – Sep. 2002 / Apr. 2005)
A. 140 lbs
B. 142 lbs
C. 146 lbs
D. 144 lbs
(REE – May 2009)
5. A roller whose diameter is 20 in. weighs 72 lbs. What horizontal force is necessary to pull the roller over a brick 2 In high, when the force is applied to the center?
A. 32 lbs
B. 30 lbs
C. 26 lbs
D. 24 lbs
(REE – May 2008)
6. An object weighing 425 N is held by a rope that passes over a horizontal drum. The coefficient of friction between the rope and the drum is 0.27. If the angle of contract is 160 deg. determine the force that will raise the object.
A. 871 N
B. 851 N
C. 881 N
D. 861 N
7. A car travels 30 km east and then 40 km north or a total distance of 70 km in a time of one hour.
A. What is the average speed?
A. 40kph
B. 50 kph
C. 60 kph
D. 70 kph
B. What is the average velocity?
A. 50 kph, N 53.13 deg E
B. 50 kph, E 53.13 deg N
C. 70 kph, N 53.13 deg E
D. 70 kph, E 53.13 deg N
(REE – Oct. 1996)
8. An automobile moving at a constant velocity of 15 m/sec passes a gasoline station. Two seconds later. another automobile leaves the gasoline station and accelerates at a constant rate of 2 m/sec sq. How soon will the second automobile overtake the first?
A. 15.3 sec
B. 16.8 sec.
C. 13.5 sec
D. 18.6 sec.
(REE – Apr. 2004)
9. Two piers A and B are located on a river, one mile apart. Two men must make round trips from pier A to pier B and return. One man is to row a boat at a velocity of 4 mi/hr relative to the water and the other man is to walk on the shore at a velocity of 4 mi/hr. The velocity of the river is 2 mi/hr in the direction from A to B. How long does it take each man to make the roundtrip?
A. 0.67 hr, 0.6 hr
B. 0.5 hr, 0.46 hr
C. 0.5 hr, 0.3 hr
D. 0.67 hr, 0.5 hr
(REE – Sep. 2007)
10. A stone is dropped from the top of a tall building and 1 sec later a second stone is thrown vertically down with a velocity of 60 ft/sec. How far below the top of the building will the second stone overtake the first?
A. 35.5 ft
B. 42.6 ft
C. 39.4 ft
D. 28.7 ft
(REE – Apr. 2007)
11. A student determine to test the law of gravity for himself walks off a skyscraper 900 ft high, stopwatch in hand and stars his free fall (zero initial velocty). Five seconds later, Superman arrives at the scene and dives off the roof to save the student. What must Superman’s initial velocity be in order that he catch the student just before the ground is reached? (Assume that Superman’s acceleration is that of any freely falling body).
A. 230 fps
B. 400 fps
C. 320 fps
D. 240 fps
(REE – Oct. 2000)
12. A student determined to test the law of gravity for he walks off a skyscraper stopwatch in hand, and starts his free fall (zero initial velocity). Five second later. Superman arrives at the scene and dives off the roof to save the student. What must be the height of the skyscraper so that even Superman can’t save him? (Assume that Superman’s acceleration is that of any freely falling body)
A. 200 ft
B. 300 ft
C. 400 ft
D. 500 ft
13. Water drips from a faucet at a uniform rate of 4 drops/second. Find the distance between two successive drops if the upper drop has been in motion for 3/8 second.
A. 0.613125 m
B. 1.22625 m
C. 1.916 m
D. 3.83 m
14. A stone is dropped from a cliff into the ocean. The sound of the impact of the stone on the ocean surface is heard 6.5 sec after it is dropped. The velocity of sound is 340 m/sec. How high is the cliff?
A. 22.10 m
B. 13.25 m
C. 175.61 m
D. 47.01 m
REE – Oct. 2000/ Apr. 2004
15. A ballon rising vertically with a velocity of 16 ft/sec, releases a sandbag at an instant when the balloon is 64 ft. above the ground. Compute the position of the sandbag 1/4 sec. after its release.
A. 5 ft
B. 3 ft
C. 1 ft
D. 2 ft
(REE – Apr. 2001/Sep. 2001)
16. A golf ball is driven horizontally from an elevated tee with a velocity of 80 ft/sec it strikes the fairway 2.5 sec. later. How far has it traveled horizontally?
A. 202 ft
B. 198 ft
C. 200 ft
D. 205 ft
(REE – Sep. 2005)
17. A golf ball is driven horizontally from an elevated tee with a velocty of 80 ft/sec it strikes the fairway 2.5 sec. later. How far has it traveled vertically?
A. 105 ft
B. 120 ft
C. 100 ft
D. 130 ft
(REE – Sep. 2008)
18. A Filipino football player kicks a football at an angle of 37 deg with the horizontal and with an initial velocity of 48 ft/sec. A second player standing at a distance of 100 ft from the first in the direction of the kicks starts running to meet the ball at the instant it is kicked. How fast must he run in order to catch the ball before it hits the ground?
A. 18.22 ft/sec
B. 17.22 ft/sec
C. 16.28 ft/sec
D. 19.46 ft/sec
19. A man who can throw a stone with a velocity of 25 m/sec wishes to hit a target T placed on his own level at a distance of 30 m. Neglecting the effects of air resistance, at what angle to the horizontal should he throw the stone?
A. 25 deg
B. 50 deg
C. 76 deg
D. 87 deg
20. A plane is flying horizontally 350 km per hour at an altitude of 420 m. At this instant, a bomb is released. How far horizontally from this point will the bomb hits the ground.
A. 785 m
B. 900 m
C. 625 m
D. 577 m